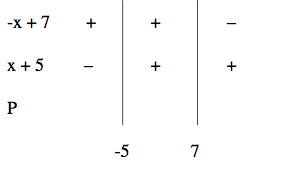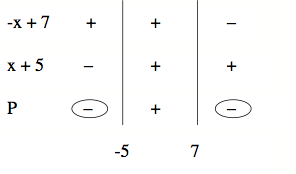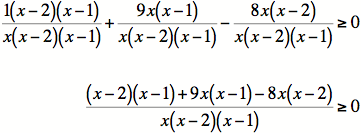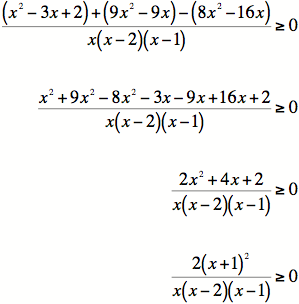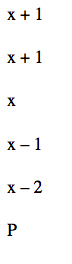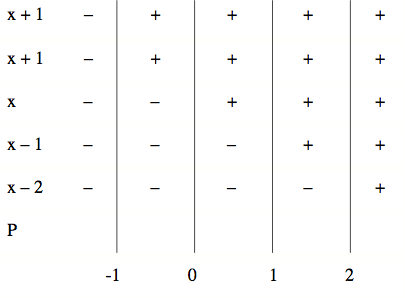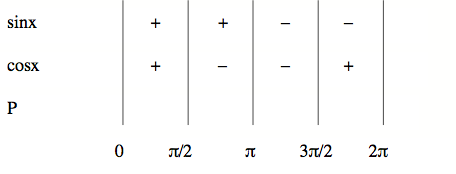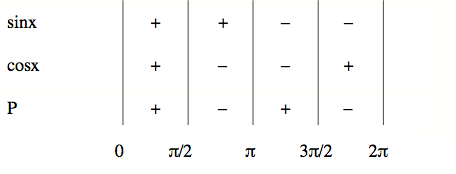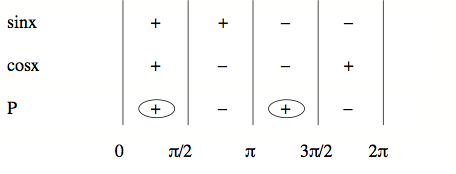Calculus Methods
02 Solving Polynomial Inequalities
1) Get all parts of the inequality on one side of the
equation, leaving 0 on the other side.
2) Gather all terms into a single fraction.
3) Factor both the numerator and denominator.
4) Create a "sign chart" to determine when each
factor is positive and when it is negative.
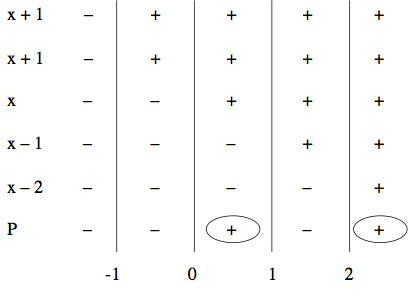
A) List all factors in a single column on the
left, and add a last row named "P" (for
product).
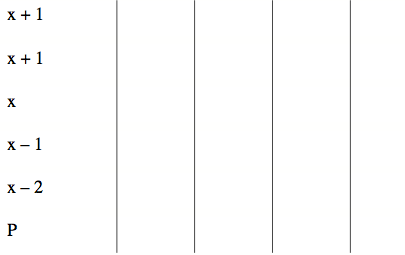
B) Draw a vertical line for each distinct zero
of each factor.
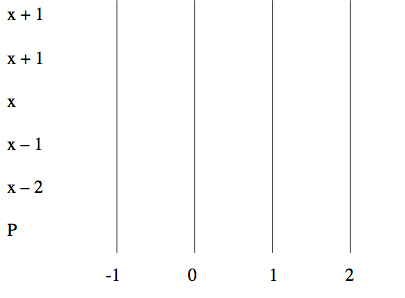
C) At the bottom of each line, write the
zeroes (roots) down in increasing numerical
order. These numbers form a number line.
D) For each row (each factor), write a "+" or
"-" to indicate whether the given factor is
positive or negative on the intervals between
successive zeroes.
E) For the "P" row, simply multiply all of the
+s and -s together to find out whether the
total product is positive or negative on that
interval.
5) Check the factored inequality that you obtained
in step (3). Are you looking for the positive
products (greater than 0) or the negative parts (less
than 0)? Also, determine when given endpoints
(zeroes) can be included, such as "less than or equal
to", and when they cannot, such as "less than" and
zeroes in the denominator. Infinity is NEVER
included IN a domain! It is not a number! Write
down your final interval(s).
COMMON PITFALL: Make sure that all terms are
on one side and 0 is on the other. Since we are
measuring when factors are positive or negative, we
need to be comparing the factors to zero.
COMMON PITFALL: Make sure to include an
overall negative constant, assuming there is one. It
is always negative in each interval, and that will
change the overall sign of that interval. To avoid
having to include this, multiply both sides of your
final equation by -1 (make sure to change the
direction of the inequality!) to rid yourself of the
negative term.
TIP: Factors change sign at their own zeros, so it's
not necessary to check every single interval for
every single factor. Just figure out which zero or
zeroes belong to a particular factor and determine
the sign on one interval. From there simply make
sure to change signs at its zero or zeroes.
TIP: Factors that have even powers do not need to
be included in a sign chart since all of the
occurrences of that factor will always net a positive
result.
TIP: Factors that have odd powers only need to be
listed once (see above tip).
Example
#1: Find all solutions of the inequality:
![]() .
.
1)
![]()
2) It is already a single fraction (denominator of 1!).
3)
![]()
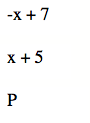 4A)
4A)

4B)
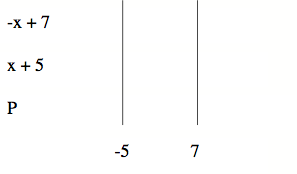 4C)
4C)
4D)